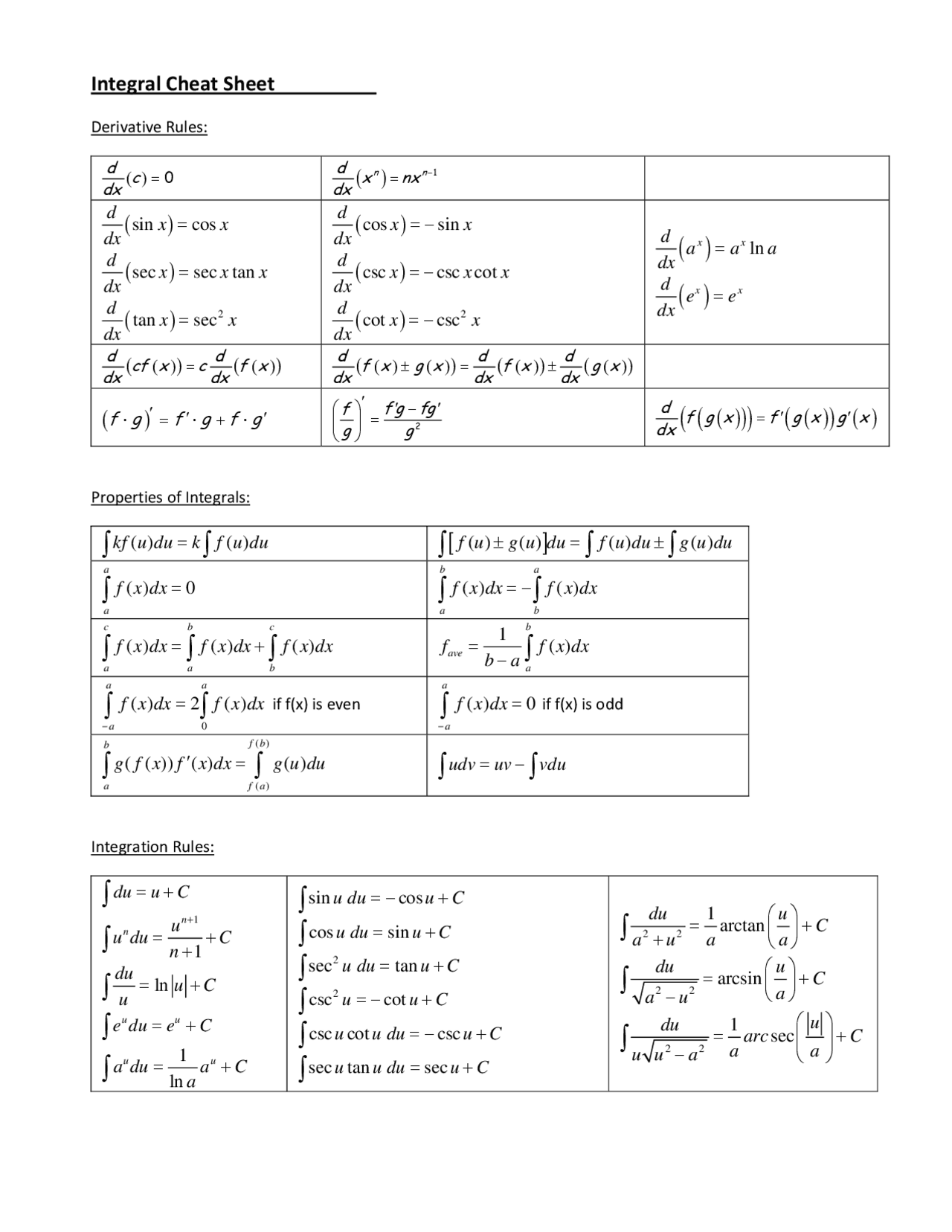
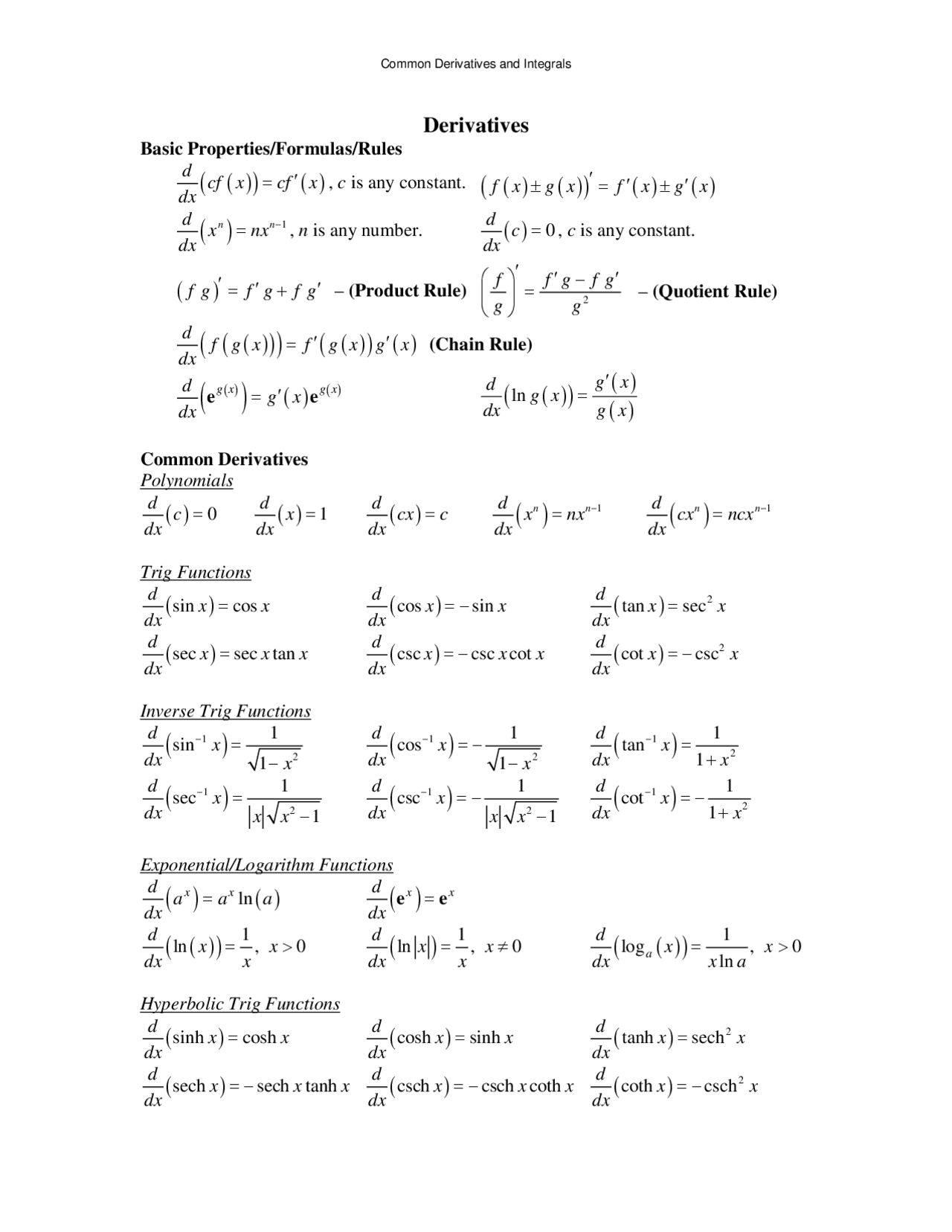
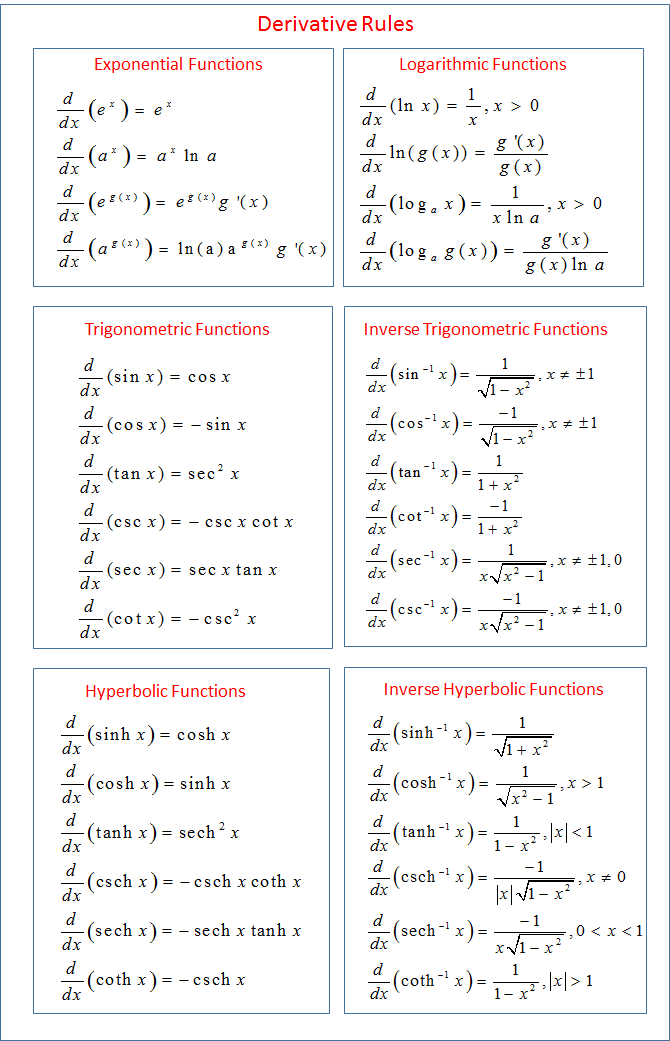
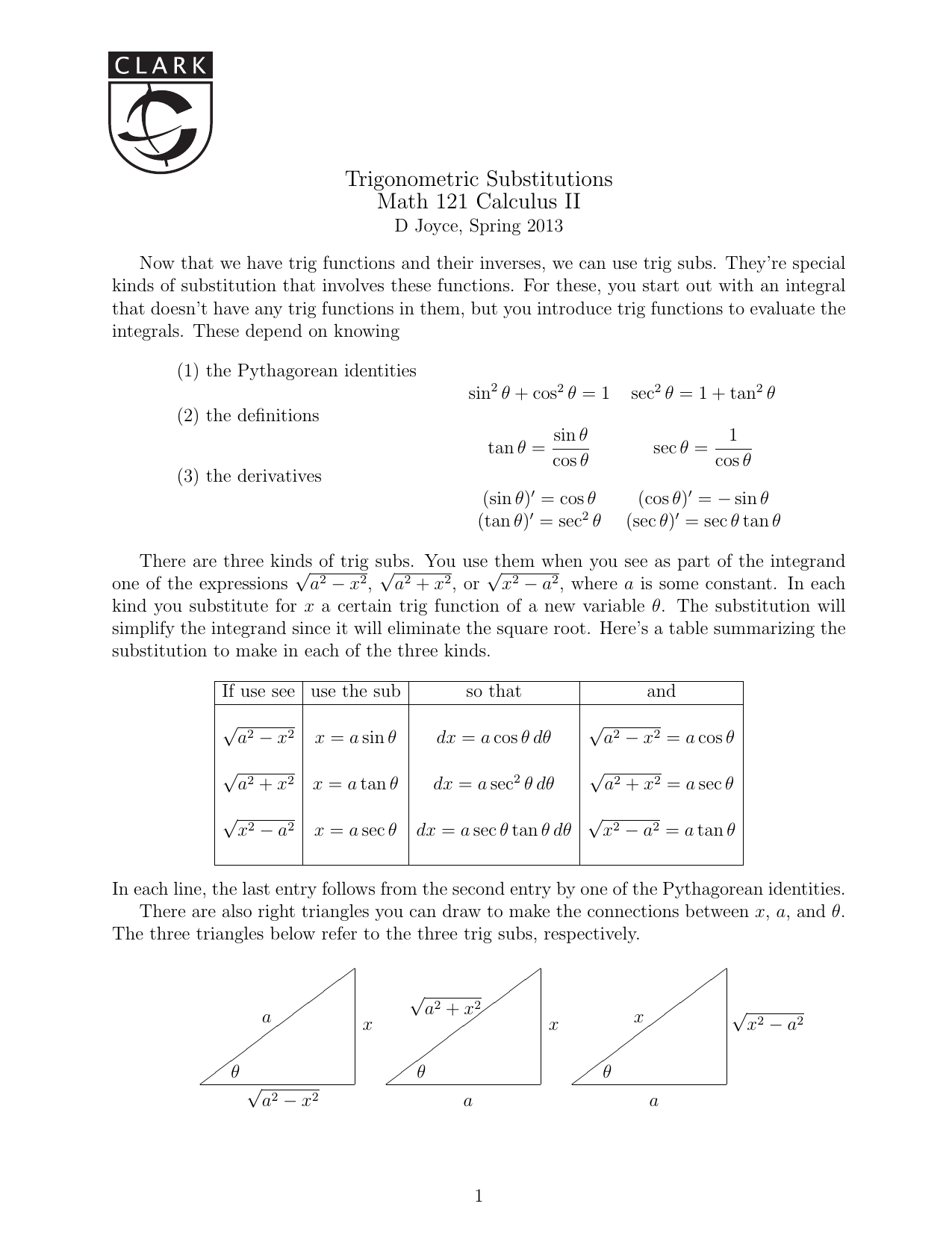
Derivatives And Integrals Cheat Sheet
Derivatives And Integrals Cheat Sheet - F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. Decomposition according to the following. D dx c = 0,cisanyconstant. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. For each factor in the denominator we get term(s) in the. Web fraction decomposition of the rational expression.
Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. Decomposition according to the following. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. D dx c = 0,cisanyconstant. Web fraction decomposition of the rational expression. For each factor in the denominator we get term(s) in the.
Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. For each factor in the denominator we get term(s) in the. Web fraction decomposition of the rational expression. Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. Decomposition according to the following. D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). D dx c = 0,cisanyconstant.
Integral cheat sheet Docsity
Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. D dx c = 0,cisanyconstant. Decomposition according to the following. For each factor in the denominator we get term(s) in the. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'.
Derivative and Integral Cheat Sheet Cheat Sheet Calculus Docsity
Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. Decomposition according to the following. For each factor in the denominator we get term(s) in the.
Image result for calculus derivatives list Differentiation Formulas
Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. Web fraction decomposition of the rational expression. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber.
Integral Cheat Sheet Calculus derivative calc trig hyperbolic integral
D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. D dx c = 0,cisanyconstant. Web fraction decomposition of the rational expression. Decomposition according to the following. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'.
Mathematics Derivatives and Integrals Chart PDF
Decomposition according to the following. Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. Web fraction decomposition of the rational expression. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. D dx c = 0,cisanyconstant.
Common Integrals And Derivatives Pdf slidesharedocs
Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. Decomposition according to the following. Web fraction decomposition of the rational expression. D dx c = 0,cisanyconstant. For each factor in the denominator we get term(s) in the.
UPD Samplexes, Readings, and Materials UPD Derivatives and Integrals
D dx c = 0,cisanyconstant. Web fraction decomposition of the rational expression. For each factor in the denominator we get term(s) in the. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). Decomposition according to the following.
Calculus Derivative Rules (video lessons, examples, solutions)
Web fraction decomposition of the rational expression. D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. For each factor in the denominator we get term(s) in the. Decomposition according to the following.
Trig integrals and derivatives cheat sheet asevdj
D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber. For each factor in the denominator we get term(s) in the. Web fraction decomposition of the rational expression. Decomposition according to the following. D dx c = 0,cisanyconstant.
UPD Samplexes, Readings, and Materials UPD Derivatives and Integrals
Sum difference rule \left (f\pm g\right)^'=f^'\pm g^'. D dx c = 0,cisanyconstant. Web derivatives basicproperties/formulas/rules d dx cf(x) = cf0(x),cisanyconstant. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). Decomposition according to the following.
Web Derivatives Basicproperties/Formulas/Rules D Dx Cf(X) = Cf0(X),Cisanyconstant.
Web fraction decomposition of the rational expression. Decomposition according to the following. F(x)g(x) 0 = f 0(x)g(x)+f(x)g0(x). D dx f(x) g(x) = f0(x) g0(x) d dx xn = nxn 1,nisanynumber.
Sum Difference Rule \Left (F\Pm G\Right)^'=F^'\Pm G^'.
D dx c = 0,cisanyconstant. For each factor in the denominator we get term(s) in the.